Best NCERT Solutions for Class 10 Science Chapter 9 Light Reflection and Refraction

NCERT Solutions for Class 10 Science Chapter 9-Light Reflection and Refraction

Best NCERT Solutions for Class 10 Science Chapter 9 Light Reflection and Refraction .A vital component of our everyday lives, light influences our perception of the environment. The two main characteristics of light are refraction and reflection, which are essential to many technical applications as well as natural events.

1. What is the principal focus of a concave mirror?

Answer: After reflecting off the concave mirror, the light beams parallel to the principal axis converge at a point on the principal axis.

The principal focus of a concave mirror is the place where the principal axis of the mirror converges.

2. What is the focal length of a spherical mirror whose radius of curvature is $20 c m$ ?

Answer: Considering that,

A spherical mirror’s radius of curvature, R=20cm
The radius of curvature is known to be twice the focal length.
R = 2f ⇒f = R/2 ⇒f = 202 ⇒f = 10 cm

Consequently, a spherical mirror with a 20 cm radius of curvature for its focal length

is equal to 10 cm.

3. Which mirror gives an erect and enlarged image of an object?

Answer: An erect, imaginary picture is created when an object is positioned between a concave mirror’s pole and principal focus.

Convex or plane mirrors do not allow for erect or expanded pictures.

4. Why is convex mirror preferred as a rear-view mirror in vehicles?

Answer: mAn erect and decreased picture is created when objects are positioned in front of a convex mirror.

We require erect images in cars and the ability to view as much of the surroundings behind them as we can.

Thus, when it comes to rearview mirrors in cars, convex mirrors are recommended.

5. What is the focal length of a convex mirror whose radius of curvature is $32 c m$ ?

Answer: Considering that,

A convex mirror’s radius of curvature, R=32 cm

As is well known,

The focal length is twice the radius of curvature.

R = 2f ⇒f = R/2 ⇒f = 322 ⇒f = 16 cm

Consequently, the convex mirror’s focal length with a curvature radius of 32 cm

is equal to 16 cm.

6. Find the location of image for a concave mirror that produces three times magnified (enlarged) real image of the object placed at $10 c m$ in front of it.

Answer:

Considering that,

The object’s distance in front of the mirror, u=−10cm

(Down indication because of the object’s placement in front of the mirror)
Image distance from mirror, v=?
As is well known,
An enlarged spherical mirror,m=hiho=−vu
location, how tall is the picture?
What is the object’s height?
Assume that ho=h.
It is stated that the object’s actual expanded image is created three times.
Thus, because of actual picture development, hi=−3h.
⇒m=−3hh=−vu

⇒3=v−10 ⇒v=−30cm

(negative sign due to the development of inverted picture)
Therefore, the placement of image from the mirror is at a distance of 30cm

and the image’s essence is reversed.

7. When a light ray travelling in the air enters obliquely into the water, how does it bend towards the normal or away from the normal? State reason.

Answer:

A light beam refracts towards the normal when it moves from a rarer to a denser material.

Because the light ray is transitioning from an air-like (rarer) medium to a water-like (denser) medium, it bends in this direction towards normal.

8. Find the speed of light in glass if the light enters from air to glass having a refractive index of $1.50$ . Take the speed of light in vacuum, $c = 3 \times 10^{8} m s^{- 1}$ .

Answer:

Considering that,

Glass’s refractive index, μ=1.5

The vacuum speed of light, c=3108ms1,
The glass’s light’s speed, vg=?
As is well known, μ=cvg⇒1.5=3×108ms−1vg.

⇒vg=2×108ms−1 ⇒vg=3×1081.5
Consequently, vg=2×108ms−1 is the speed of light in glass.

9. From the table, find the medium having the highest optical density and the lowest optical density.

Material medium	Refractive Index	Material medium	Refractive Index
Air	1.0003	Crown glass	1.52
Ice	1.31	Canada Balsam	1.53
Water	1.33	Rock salt	1.54
Alcohol	1.36	Carbon disulphide	1.63
Kerosene	1.44	Dense flint glass	1.65
Fused quartz	1.46	Ruby	1.71
Turpentine oil	1.47	Sapphire	1.77
Benzene	1.50	Diamond	2.42

Answer : Check the material’s refractive index to determine which have the highest and lowest optical densities. The optical density of the two will be highest for the one with the highest refractive index and lowest for the one with the lowest.

Diamond has the maximum optical density, or ϼ=2.42.

Air has the lowest optical density, or μ=1.0003.

10.Among kerosene, turpentine oil and water in which medium does the light travel fastest? Refer to the table for refractive index.

Material Medium	Refractive Index	Material Medium	Refractive Index
Air	1.0003	Crown Glass	1.52
Ice	1.31	Canada Balsam	1.53
Water	1.33	Rock Salt	1.54
Alcohol	1.36	Carbon Disulphide	1.63
Kerosene	1.44	Dense Flint Glass	1.65
Fused Quartz	1.46	Ruby	1.71
Turpentine Oil	1.47	Sapphire	1.77
Benzene	1.50	Diamond	2.42

Answer: As is well known,

Absolute refractive index,μ=cvm

where vm is the speed of light in medium and is the speed of light in vacuum, calculated as 3108ms1vm.

(The constant is c)

We compare the refractive index in order to compare the speed of light. Refractive index and light speed are inversely correlated, meaning that a higher refractive index corresponds to a slower speed of light.

Kerosene, turpentine oil, and water have the following refractive index orders: μkerosene>μturpentineoil>μwater.

The speed of light is now in the following order: kerosene, turpentine oil, water.

Light travels at the fastest speed in water as a result.

11. What is the meaning of the statement “The refractive index of diamond is $2.42$ ”?

Answer:

As is well known,

Refractive index of a medium,μ=cvm

where “The refractive index of diamond is 2.42.” where “c” is the speed of light in a vacuum and “vm” is the speed of light in a medium.

indicates that 2.42 is the speed of light in a vacuum.

12.42 is the product of the speed of light in diamond times its speed.

times the vacuum speed of light.

12. What is $1$ dioptre of power of a lens?

Answer:

P equals 1 f(meters) where f is the focal length in meters of a lens with power P.

The dioptre, represented by D, is the S.I. unit of power for a lens.
The focal length of a lens with one power

One dioptre is equal to one metre.

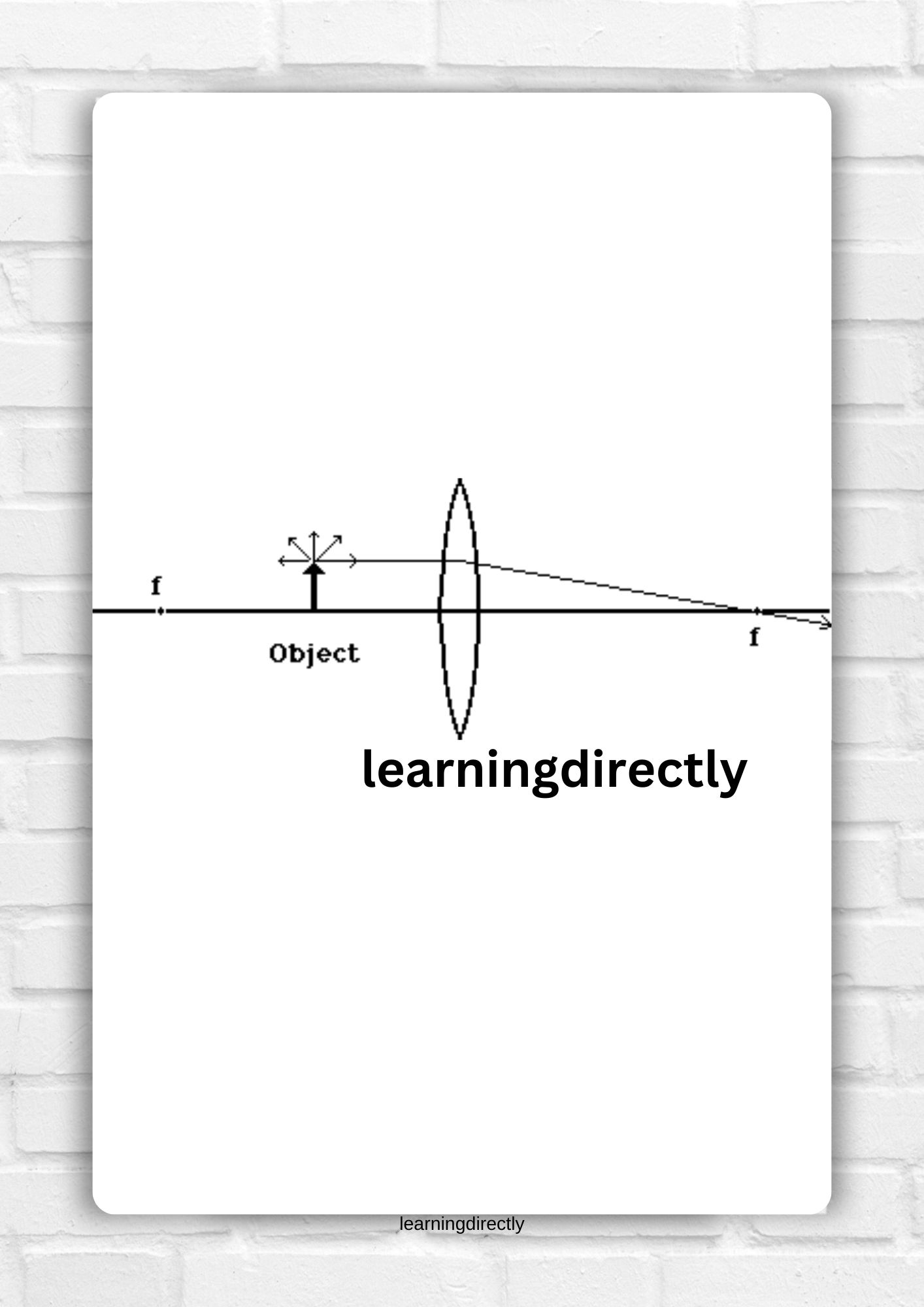
13. Image formed by a convex lens is real and inverted and at a distance of $50 c m$ from the lens. Find the position of the needle in front of the convex lens when the image is equal to the size of the object. Also, calculate the power of the lens.

Answer:

Considering that,

Image distance from convex lens, v = 50 cm

The object’s distance in front of the lens, u=?

The resulting image is both real and reversed. Thus, the lens has a magnification of −1.

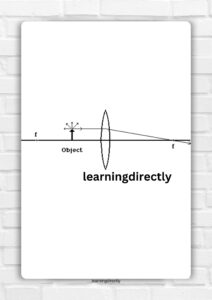
$Best NCERT Solutions for Class 10 Science Chapter 9 Light Reflection and Refraction$

As is well known,

A convex lens magnified, m=vu⇒−1=vu⇒−1=50u⇒u=−50c
Lens equation: 1v − 1u = 1f

is the lens’s focal length.

⇒1f=150−1(-50)

→1f=150+150

→1f=250

⇒f=502

⇒f=25cm=0.25m

It is established that P=1f(meters) is the lens’s power.

⇒P=1(+0.25) ⇒P=+4D
Consequently, u=−50cm is the object’s distance from the lens.

and the lens’s power is P=+4D.

14. What is the power of a concave lens of focal length $2 m$ ?

Answer:

Considering that,

A concave lens’s focal length is f = −2m.

Concave lens power, P=1f(meters) ⇒P=1(−2) ⇒P=−0.5D
Consequently, P=−0.5D is the concave lens’s power.

15.Which one of the following materials cannot be used to make a lens?

Water

Glass

Plastic

Clay

Answer: d) Clay can’t be used to make a lens because it is opaque.

16.The image formed by a concave mirror is observed to be virtual, erect and larger than the object. Where should the position of the object be?

Between the principal focus and the centre of curvature

At the centre of curvature

Beyond the centre of curvature

Between the pole of the mirror and its principal focus.

Answer: d) When an object forms a virtual, erect picture that is larger than the object in the concave mirror, it is positioned between the mirror’s pole and principal focus.

17. Where should an object be placed in front of a convex lens to get a real image of the size of the object?

At the principal focus of the lens
At twice the focal length
At infinity
Between the optical centre of the lens and its principal focus

Answer:

To acquire an accurate representation of an object’s size, it should be positioned twice as far away as the convex lens’s focal length.

18. A spherical mirror and a thin spherical lens have each a focal length of $- 15 c m$ . The mirror and the lens are likely to be

Both concave
Both convex
The mirror is concave and the lens is convex
The mirror is convex, but the lens is concave

Answer: a) A concave lens’s primary focus is negative and located on the same side as the object. The focus is negative and in front of the mirror in the case of a concave mirror. As a result, it’s likely that the lens and mirror are concave.

19. No matter how far you stand from a mirror, your image appears erect. The mirror is likely to be

Plane
Concave
Convex
Either plane or convex

Answer: d) Both convex and flat mirrors can create erect images of things in any position.

20. Which of the following lenses would you prefer to use while reading small letters found in a dictionary?

A convex lens of focal length
$50 c m$
A concave lens of focal length
$50 c m$
A convex lens of focal length
$5 c m$
A concave lens of focal length
$5 c m$

Answer: a)Magnified and erect pictures are created in a convex lens when an object is positioned between the optic centre and the focus. Therefore, it is better to use a convex lens when reading small lettering.

21. We wish to obtain an erect image of an object, using a concave mirror of focal length

15 c m

. What should be the range of distance of the object from the mirror? What is the nature of the image? Is the image larger or smaller than the object? Draw a ray diagram to show the image formation in this case.

Answer: The object needs to be positioned between the focus and the optic centre of a concave mirror in order to produce an erect image.

The concave mirror’s focal length is specified as 15 cm in this instance.
As a result, the object’s range of distance from the mirror is 0 cm.

up to 15 cm

The image is virtual in nature.

The object is smaller than the photograph.

22. Name the type of mirror used in the following situations and support your answer with reason.

Headlights of a car

Answer: An automobile’s concave mirror is utilised in its headlights. Because if the lightbulb is positioned at the focus of a concave mirror, a parallel beam of light is produced.

Side/Rear-View Mirror of a Vehicle

Answer: A convex mirror is utilised in a car’s side or rearview mirror. Since erect and diminished pictures are generated when things are put in front of a convex mirror, a broader range of view is provided.

Solar Furnace

Answer: Solar furnaces employ concave mirrors. Because of their converging qualities, they converge sunlight to a point and raise temperatures.

23. One-half of a convex lens is covered with a black paper. Will this lens produce a complete image of the object? Verify your answer experimentally. Explain your observations.

Answer: Yes, a fuller, less intense image of the object is produced by the lens.

24. An object of

5 c m

in length is held

25 c m

away from a converging lens of focal length

10 c m

. Draw the ray diagram and find the position, size and the nature of the image formed.

Answer:

Considering that,

The object’s height, ho=5 cm
The object’s distance in front of the lens, u=−25cm
Image distance from lens, v=?

Lens focal length, f=+10cm

Lens formula: 1v − 1u = 1f

⇒1v=1f+1u

⇒1v=110−125

→1v = 25 -10250 →1v = 15250

⇒v=25015 ⇒v=16.66 cm

The amount that v is positive

shows that the item and the image are on different sides of each other.
It is well known that m=hiho=−vu for magnification
Height of the image, hi=? ⇒m=hi5=−16.6625=−0.66 ⇒hi=−0.66×5 ⇒hi=−3.3cm

Given the magnification of -0.66, the object is inverted, as indicated by the negative sign, and the image is smaller than the object when the magnification is less than 1.

Consequently, the image’s location is 16.66 cm.

through the lens. The piece is 3.3 centimetres in height.

The image’s nature is decreased, distorted, and real.

25. A concave lens of focal length

15 c m

forms an image

10 c m

from the lens. Find the distance of an object from the lens? Draw the ray diagram.

Answer:

Considering that,

The lens’s focal length is f = -15 cm.
Image distance from lens, v = −10cm
The object’s distance in front of the lens, u=?
Lens formula: 1v − 1u = 1f

⇒1u=1v−1f

⇒1u=1(−10)−1(−15)

⇒1u=−110+115

⇒1u=-5150 →u=-1505 ⇒u=-30cm

Thus, the object is at a distance of $30 c m$ from the lens.

26. An object is placed at a distance of

10 c m

from a convex mirror of focal length

15 c m

. Find the position and nature of the image.

Answer:

Considering that,

The convex mirror’s focal length, f=+15cm

The object’s distance in front of the convex mirror, u=−10cm
The image’s distance from the convex mirror, v=?
A mirror formula yields 1v+1u=1f, therefore 1v=1f−1u.

→ 1v=115−1(−10) ⇒ 1v=115+110 ⇒ 1v=25150 ⇒ v=15025 ⇒ v=6cm
The amount that v is positive

shows that the picture is virtual and produced behind the mirror.
Magnification is known to be m=hiho=−vu⇒m=−6−10=+0.6
The image is erect, as shown by the positive magnification. Since the magnification is not one,

It shows that the thing is larger than the image.

As a result, the image’s location is 6 cm.

in front of the mirror. The image’s nature is shrunken, erect, and imaginary.

27. What does “The magnification produced by a plane mirror is

+ 1

” mean?

Answer:

As is well known,

A plane mirror magnifies an object by m=hiho=−vu.
location, how tall is the picture?

What is the object’s height?
u is the object’s distance in front of the lens.

v is the image’s distance from the lens.

A plane mirror produces a +1 magnification.

implies that hi=ho

that is, the object’s size and the image’s size are equal. The image is erect, as indicated by the positive size.

Magnification is therefore equivalent to +1.

indicates that the image’s size is equal to that of the item and erect.

28. An object

5 c m

in length is placed at a distance of

20 c m

in front of a convex mirror of radius of curvature

30 c m

. Find the position of the image, its nature and size.

Answer:

Considering that,

The object’s distance in front of the mirror, u=−20cm

Image distance from the mirror, v=?

The mirror’s radius of curvature, R=30 cm

The mirror’s focal length, f=?

As is well known,

Twice the focal length equals the radius of curvature.

R=2f, 30=2f, and f=302=15 cm
The mirror formula is as follows: 1v+1u=1f, 1v=1f−1u, 1v=115−1(−20), 1v=115+120, and 1v=20+15300.

→1v=35300

⇒v=607 ⇒v=8.57cm

The amount that v is positive

shows that the mirror is the source of the image.

As is well known,

Enlargement, m=hiho=−vu

The object’s height, ho=5 cm

Height of the image, hi=? ⇒hi=−vu×ho

⇒hi=-8.57(-20)×5

⇒hi = 2.14 cm

As a result, 8.57 cm is the distance at which the image is produced.

in front of the mirror. The image has a reduced, erect, and virtual quality.

29. An object of size

7.0 c m

is placed at

27 c m

in front of a concave mirror of focal length

18 c m

. At what distance from the mirror should a screen be placed, so that a sharp focused image can be obtained? Find the size and the nature of the image.

Answer:

Considering that,

The object’s distance in front of the mirror, u = -27 cm

Image distance from the mirror, v=?

Mirror focal length, f = -18 cm

1v+1u=1f is the mirror formula.

⇒1v=1f−1u

→1v=−18−1(−27) ⇒1v=−118+127
→1v=−3+254
⇒1v=−154

⇒v=-54 cm
The negative amount that v

suggests that the screen should be set back 54 centimetres.

front the mirror, and the picture is accurate.

It is well known that m=hiho=−vu for magnification
Height of the object, ho=7cm Height of the image, hi=?

⇒hi=−vu×ho
Hi = -54(-27)×7; hi = -14cm

The image is 14 cm tall.
Therefore, the image is formed at a distance of 54cm

front the mirror. The image’s nature is actual, magnified, and inverted.

30. Find the focal length of a lens of power

- 2.0 D

. What type of lens is this?

Answer:

Considering that,

Lens power, P = -2.0D

A lens’s focal length, f=?

Lens power, P=1f(meters)

Negative f=−2=1f ⇒f=−12=−0.5m

suggests a concave lens.
Consequently, the lens’s focal length is f=−0.5m.

the lens has a concave shape.

31. A doctor has prescribed a corrective lens of power $+ 1.5 D$ . Find the focal length of the lens. Is the prescribed lens diverging or converging?

Answer:

Considering that,

Lens power, P=+1.5D

A lens’s focal length, f=?

Lens power: P = 1f(meters) ⇒1.5 = 1f ⇒f = 11.5 = 0.66 m
A convex lens is indicated by a positive f.

Consequently, f=0.66m is the focal length of the lens.

and the lens prescribed is a divergent lens.